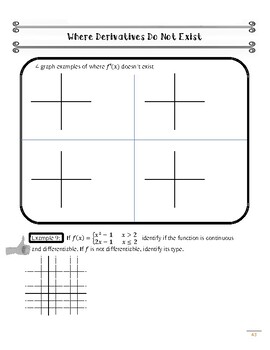
- Unit 3 Basic Differentiationap Calculus 14th Edition
- Unit 3 Basic Differentiationap Calculus Calculator
- Unit 3 Basic Differentiationap Calculus Solver
- Notes 3.1 Key Notes 3.2 Key Notes 3.3 Key Smac Edition Notes 3.3 Key Notes 3.4 Key Notes 3.5 Key.
- BASIC CONCEPTS OF DIFFERENTIAL AND INTEGRAL CALCULUS CHAPTER 8 Integral Calculus Differential Calculus Methods of Substitution Basic Formulas Basic Laws of Differentiation Some Standard Results Calculus After reading this chapter, students will be able to understand: Understand the basics of differentiation and integration.
More Videos
Calculus is a branch of mathematics that focuses on the calculation of the instantaneous rate of change (differentiation) and the sum of infinitely small pieces to determine the object as a whole (integration). Two popular mathematicians Newton and Gottfried Wilhelm Leibniz developed the concept of calculus in the 17th century.
Unit 1: Function & Trig Review. Unit 2: Limits & Continuity. Unit 3: Differentiation. Unit 3 - Basic Derivative Rules Notes.pdf (125k) Unknown user. Implicit differentiation is very similar to normal differentiation, but every time we take a derivative with respect t, we need to multiply the result by We also differentiate the entire equation from left to right, including any numbers.
Today, this is the basic entry point for students who want to learn chemistry, physics, science, economics, or finance etc. If you wanted to calculate the position of the space shuttle from shuttle then it is possible through calculus even the problem is complex or quite difficult. These days there are a plenty of computer tools that can be used to solve the calculus problem in minutes.
What is Differentiation in Calculus?
The process of finding derivatives or instantaneous rate of change with respect to a function, it is termed as differentiation. In contrast, this is possible to carry out differentiation by real algebraic manipulations, rules of operations, and the information on how to manipulate functions.
Differentiation is a technique to measure the rate of change for curves, graphs, images, etc. You can determine the tangent or slope along a given direction.With this process, you can also check where the lower and upper values occur. The early applications of differentiation in calculus include planetary motion, gravity, ship design, fluid flow, geometrical shapes, and bridge engineering etc.
List of Basic Differentiation Formulas
Take an example of the small curve whose slope or tangent is difficult to calculate without the right technique. Here, we had to use a list of basic differentiation formulas to make the process easier. You can also calculate the average rate of change over the longer time intervals where actual speed is difficult to determine without proper formulas.
Partial Differentiation Calculus Formulas
Partial derivatives in the mathematics of a function of multiple variables are its derivatives with respect to those variables. Partial derivatives are used for vectors and many other things like space, motion, differential geometry etc.
Differentiation Calculus Rules
The derivatives are used to calculate the slope of a function at any given point. There are differentiation rules that can be used in different conditions as per the requirement. They are tough to understand at a first glance, So, you need a proper understanding of the rules before you actually implement them for the complex problems.
Why do students need to learn Differentiation Formulas?
The applications of derivatives in real-life are just the endless and they can be utilized in almost every sector like physics, chemical engineering, science, space, differential geometry, ship design, fluid flow, bridge manufacturing, and many more. These are some practical examples where differentiation formulas are needed to calculate the slope or tangent of a function.
Other than this, differentiation formulas can also be used for the preparation of competitive exams, and higher studies. They are taken an important part of the curriculum and need continuous practice to solve tough problems. They sound difficult if you don't any in-depth understanding of differentiation formulas. SO, they are necessary to learn by students during schools and colleges etc.
Related posts:
Section 5-1 : Basic Concepts
Let's start this section off with a quick discussion on what vectors are used for. Vectors are used to represent quantities that have both a magnitude and a direction. Good examples of quantities that can be represented by vectors are force and velocity. Both of these have a direction and a magnitude.
Let's consider force for a second. A force of say 5 Newtons that is applied in a particular direction can be applied at any point in space. In other words, the point where we apply the force does not change the force itself. Forces are independent of the point of application. To define a force all we need to know is the magnitude of the force and the direction that the force is applied in.
The same idea holds more generally with vectors. Vectors only impart magnitude and direction. They don't impart any information about where the quantity is applied. This is an important idea to always remember in the study of vectors.
In a graphical sense vectors are represented by directed line segments. The length of the line segment is the magnitude of the vector and the direction of the line segment is the direction of the vector. However, because vectors don't impart any information about where the quantity is applied any directed line segment with the same length and direction will represent the same vector.
Consider the sketch below.
Each of the directed line segments in the sketch represents the same vector. In each case the vector starts at a specific point then moves 2 units to the left and 5 units up. The notation that we'll use for this vector is,
[vec v = leftlangle { - 2,5} rightrangle ]and each of the directed line segments in the sketch are called representations of the vector.
Be careful to distinguish vector notation, (leftlangle { - 2,5} rightrangle ), from the notation we use to represent coordinates of points, (left( { - 2,5} right)). The vector denotes a magnitude and a direction of a quantity while the point denotes a location in space. So don't mix the notations up!
A representation of the vector (vec v = leftlangle {{a_1},{a_2}} rightrangle ) in two dimensional space is any directed line segment, (overrightarrow {AB} ), from the point (A = left( {x,y} right)) to the point (B = left( {x + {a_1},y + {a_2}} right)). Likewise a representation of the vector (vec v = leftlangle {{a_1},{a_2},{a_3}} rightrangle ) in three dimensional space is any directed line segment, (overrightarrow {AB} ), from the point (A = left( {x,y,z} right)) to the point (B = left( {x + {a_1},y + {a_2},z + {a_3}} right)).
Note that there is very little difference between the two dimensional and three dimensional formulas above. To get from the three dimensional formula to the two dimensional formula all we did is take out the third component/coordinate. Because of this most of the formulas here are given only in their three dimensional version. If we need them in their two dimensional form we can easily modify the three dimensional form.
There is one representation of a vector that is special in some way. The representation of the vector (vec v = leftlangle {{a_1},{a_2},{a_3}} rightrangle ) that starts at the point (A = left( {0,0,0} right)) and ends at the point (B = left( {{a_1},{a_2},{a_3}} right)) is called the position vector of the point (left( {{a_1},{a_2},{a_3}} right)). So, when we talk about position vectors we are specifying the initial and final point of the vector.
Position vectors are useful if we ever need to represent a point as a vector. As we'll see there are times in which we definitely are going to want to represent points as vectors. In fact, we're going to run into topics that can only be done if we represent points as vectors.
Next, we need to discuss briefly how to generate a vector given the initial and final points of the representation. Given the two points (A = left( {{a_1},{a_2},{a_3}} right)) and (B = left( {{b_1},{b_2},{b_3}} right)) the vector with the representation (overrightarrow {AB} ) is,
[vec v = leftlangle {{b_1} - {a_1},{b_2} - {a_2},{b_3} - {a_3}} rightrangle ]Note that we have to be very careful with direction here. The vector above is the vector that starts at (A) and ends at (B). The vector that starts at (B) and ends at (A), i.e. with representation (overrightarrow {BA} ) is,
[vec w = leftlangle {{a_1} - {b_1},{a_2} - {b_2},{a_3} - {b_3}} rightrangle ]These two vectors are different and so we do need to always pay attention to what point is the starting point and what point is the ending point. When determining the vector between two points we always subtract the initial point from the terminal point.
Unit 3 Basic Differentiationap Calculus 14th Edition
Example 1 Give the vector for each of the following.- The vector from (left( {2, - 7,0} right)) to (left( {1, - 3, - 5} right)).
- The vector from (left( {1, - 3, - 5} right)) to(left( {2, - 7,0} right)).
- The position vector for (left( { - 90,4} right))
Remember that to construct this vector we subtract coordinates of the starting point from the ending point.

b The vector from (left( {1, - 3, - 5} right)) to(left( {2, - 7,0} right)).
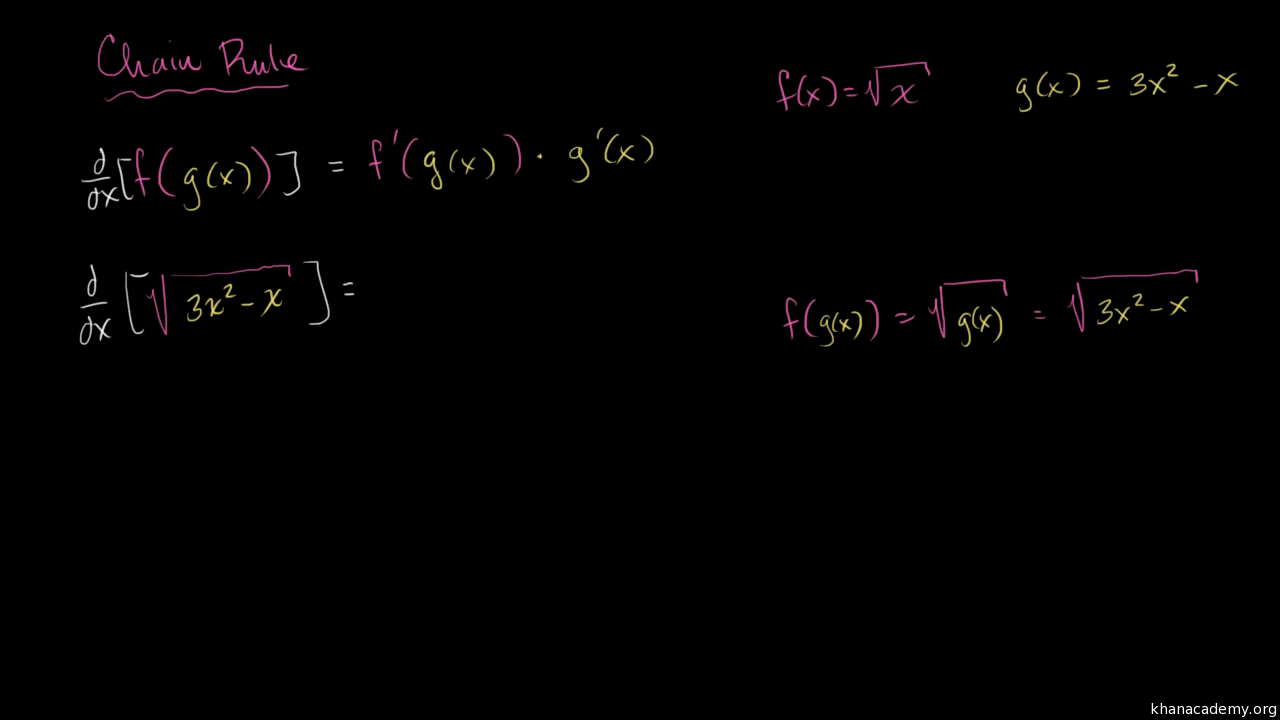
- Unit 3 Basic Differentiationap Calculus 14th Edition
- Unit 3 Basic Differentiationap Calculus Calculator
- Unit 3 Basic Differentiationap Calculus Solver
- Notes 3.1 Key Notes 3.2 Key Notes 3.3 Key Smac Edition Notes 3.3 Key Notes 3.4 Key Notes 3.5 Key.
- BASIC CONCEPTS OF DIFFERENTIAL AND INTEGRAL CALCULUS CHAPTER 8 Integral Calculus Differential Calculus Methods of Substitution Basic Formulas Basic Laws of Differentiation Some Standard Results Calculus After reading this chapter, students will be able to understand: Understand the basics of differentiation and integration.
More Videos
Calculus is a branch of mathematics that focuses on the calculation of the instantaneous rate of change (differentiation) and the sum of infinitely small pieces to determine the object as a whole (integration). Two popular mathematicians Newton and Gottfried Wilhelm Leibniz developed the concept of calculus in the 17th century.
Unit 1: Function & Trig Review. Unit 2: Limits & Continuity. Unit 3: Differentiation. Unit 3 - Basic Derivative Rules Notes.pdf (125k) Unknown user. Implicit differentiation is very similar to normal differentiation, but every time we take a derivative with respect t, we need to multiply the result by We also differentiate the entire equation from left to right, including any numbers.
Today, this is the basic entry point for students who want to learn chemistry, physics, science, economics, or finance etc. If you wanted to calculate the position of the space shuttle from shuttle then it is possible through calculus even the problem is complex or quite difficult. These days there are a plenty of computer tools that can be used to solve the calculus problem in minutes.
What is Differentiation in Calculus?
The process of finding derivatives or instantaneous rate of change with respect to a function, it is termed as differentiation. In contrast, this is possible to carry out differentiation by real algebraic manipulations, rules of operations, and the information on how to manipulate functions.
Differentiation is a technique to measure the rate of change for curves, graphs, images, etc. You can determine the tangent or slope along a given direction.With this process, you can also check where the lower and upper values occur. The early applications of differentiation in calculus include planetary motion, gravity, ship design, fluid flow, geometrical shapes, and bridge engineering etc.
List of Basic Differentiation Formulas
Take an example of the small curve whose slope or tangent is difficult to calculate without the right technique. Here, we had to use a list of basic differentiation formulas to make the process easier. You can also calculate the average rate of change over the longer time intervals where actual speed is difficult to determine without proper formulas.
Partial Differentiation Calculus Formulas
Partial derivatives in the mathematics of a function of multiple variables are its derivatives with respect to those variables. Partial derivatives are used for vectors and many other things like space, motion, differential geometry etc.
Differentiation Calculus Rules
The derivatives are used to calculate the slope of a function at any given point. There are differentiation rules that can be used in different conditions as per the requirement. They are tough to understand at a first glance, So, you need a proper understanding of the rules before you actually implement them for the complex problems.
Why do students need to learn Differentiation Formulas?
The applications of derivatives in real-life are just the endless and they can be utilized in almost every sector like physics, chemical engineering, science, space, differential geometry, ship design, fluid flow, bridge manufacturing, and many more. These are some practical examples where differentiation formulas are needed to calculate the slope or tangent of a function.
Other than this, differentiation formulas can also be used for the preparation of competitive exams, and higher studies. They are taken an important part of the curriculum and need continuous practice to solve tough problems. They sound difficult if you don't any in-depth understanding of differentiation formulas. SO, they are necessary to learn by students during schools and colleges etc.
Related posts:
Section 5-1 : Basic Concepts
Let's start this section off with a quick discussion on what vectors are used for. Vectors are used to represent quantities that have both a magnitude and a direction. Good examples of quantities that can be represented by vectors are force and velocity. Both of these have a direction and a magnitude.
Let's consider force for a second. A force of say 5 Newtons that is applied in a particular direction can be applied at any point in space. In other words, the point where we apply the force does not change the force itself. Forces are independent of the point of application. To define a force all we need to know is the magnitude of the force and the direction that the force is applied in.
The same idea holds more generally with vectors. Vectors only impart magnitude and direction. They don't impart any information about where the quantity is applied. This is an important idea to always remember in the study of vectors.
In a graphical sense vectors are represented by directed line segments. The length of the line segment is the magnitude of the vector and the direction of the line segment is the direction of the vector. However, because vectors don't impart any information about where the quantity is applied any directed line segment with the same length and direction will represent the same vector.
Consider the sketch below.
Each of the directed line segments in the sketch represents the same vector. In each case the vector starts at a specific point then moves 2 units to the left and 5 units up. The notation that we'll use for this vector is,
[vec v = leftlangle { - 2,5} rightrangle ]and each of the directed line segments in the sketch are called representations of the vector.
Be careful to distinguish vector notation, (leftlangle { - 2,5} rightrangle ), from the notation we use to represent coordinates of points, (left( { - 2,5} right)). The vector denotes a magnitude and a direction of a quantity while the point denotes a location in space. So don't mix the notations up!
A representation of the vector (vec v = leftlangle {{a_1},{a_2}} rightrangle ) in two dimensional space is any directed line segment, (overrightarrow {AB} ), from the point (A = left( {x,y} right)) to the point (B = left( {x + {a_1},y + {a_2}} right)). Likewise a representation of the vector (vec v = leftlangle {{a_1},{a_2},{a_3}} rightrangle ) in three dimensional space is any directed line segment, (overrightarrow {AB} ), from the point (A = left( {x,y,z} right)) to the point (B = left( {x + {a_1},y + {a_2},z + {a_3}} right)).
Note that there is very little difference between the two dimensional and three dimensional formulas above. To get from the three dimensional formula to the two dimensional formula all we did is take out the third component/coordinate. Because of this most of the formulas here are given only in their three dimensional version. If we need them in their two dimensional form we can easily modify the three dimensional form.
There is one representation of a vector that is special in some way. The representation of the vector (vec v = leftlangle {{a_1},{a_2},{a_3}} rightrangle ) that starts at the point (A = left( {0,0,0} right)) and ends at the point (B = left( {{a_1},{a_2},{a_3}} right)) is called the position vector of the point (left( {{a_1},{a_2},{a_3}} right)). So, when we talk about position vectors we are specifying the initial and final point of the vector.
Position vectors are useful if we ever need to represent a point as a vector. As we'll see there are times in which we definitely are going to want to represent points as vectors. In fact, we're going to run into topics that can only be done if we represent points as vectors.
Next, we need to discuss briefly how to generate a vector given the initial and final points of the representation. Given the two points (A = left( {{a_1},{a_2},{a_3}} right)) and (B = left( {{b_1},{b_2},{b_3}} right)) the vector with the representation (overrightarrow {AB} ) is,
[vec v = leftlangle {{b_1} - {a_1},{b_2} - {a_2},{b_3} - {a_3}} rightrangle ]Note that we have to be very careful with direction here. The vector above is the vector that starts at (A) and ends at (B). The vector that starts at (B) and ends at (A), i.e. with representation (overrightarrow {BA} ) is,
[vec w = leftlangle {{a_1} - {b_1},{a_2} - {b_2},{a_3} - {b_3}} rightrangle ]These two vectors are different and so we do need to always pay attention to what point is the starting point and what point is the ending point. When determining the vector between two points we always subtract the initial point from the terminal point.
Unit 3 Basic Differentiationap Calculus 14th Edition
Example 1 Give the vector for each of the following.- The vector from (left( {2, - 7,0} right)) to (left( {1, - 3, - 5} right)).
- The vector from (left( {1, - 3, - 5} right)) to(left( {2, - 7,0} right)).
- The position vector for (left( { - 90,4} right))
Remember that to construct this vector we subtract coordinates of the starting point from the ending point.
[leftlangle {1 - 2, - 3 - left( { - 7} right), - 5 - 0} rightrangle = leftlangle { - 1,4, - 5} rightrangle ]b The vector from (left( {1, - 3, - 5} right)) to(left( {2, - 7,0} right)). Show Solution
Same thing here.
[leftlangle {2 - 1, - 7 - left( { - 3} right),0 - left( { - 5} right)} rightrangle = leftlangle {1, - 4,5} rightrangle ]Notice that the only difference between the first two is the signs are all opposite. This difference is important as it is this difference that tells us that the two vectors point in opposite directions.
c The position vector for (left( { - 90,4} right)) Show Solution
Not much to this one other than acknowledging that the position vector of a point is nothing more than a vector with the point's coordinates as its components.
[leftlangle { - 90,4} rightrangle ]We now need to start discussing some of the basic concepts that we will run into on occasion.
Magnitude
The magnitude, or length, of the vector (vec v = leftlangle {{a_1},{a_2},{a_3}} rightrangle ) is given by,
[left| {overline{v}} right| = sqrt {a_1^2 + a_2^2 + a_3^2} ] Example 2 Determine the magnitude of each of the following vectors.- (vec a = leftlangle {3, - 5,10} rightrangle )
- (displaystyle vec u = leftlangle {frac{1}{{sqrt 5 }}, - frac{2}{{sqrt 5 }}} rightrangle )
- (vec w = leftlangle {0,0} rightrangle )
- (vec i = leftlangle {1,0,0} rightrangle )
There isn't too much to these other than plug into the formula.
a (left| {vec a} right| = sqrt {9 + 25 + 100} = sqrt {134} )
b (displaystyle left| {vec u} right| = sqrt {frac{1}{5} + frac{4}{5}} = sqrt 1 = 1)
c (left| {vec w} right| = sqrt {0 + 0} = 0)
d (left| {vec i} right| = sqrt {1 + 0 + 0} = 1)
We also have the following fact about the magnitude.
[{mbox{If }}left| {vec a} right| = 0{mbox{ then }}vec a = vec 0]This should make sense. Because we square all the components the only way we can get zero out of the formula was for the components to be zero in the first place.
Unit Vector
Any vector with magnitude of 1, i.e. (left| {vec u} right| = 1), is called a unit vector.
Example 3 Which of the vectors from Example 2 are unit vectors? Show SolutionBoth the second and fourth vectors had a length of 1 and so they are the only unit vectors from the first example.
Zero Vector
The vector (vec w = leftlangle {0,0} rightrangle ) that we saw in the first example is called a zero vector since its components are all zero. Zero vectors are often denoted by (vec 0). Be careful to distinguish 0 (the number) from (vec 0) (the vector). The number 0 denotes the origin in space, while the vector (vec 0) denotes a vector that has no magnitude or direction.
Standard Basis Vectors
The fourth vector from the second example, (vec i = leftlangle {1,0,0} rightrangle ), is called a standard basis vector. In three dimensional space there are three standard basis vectors,
[vec i = leftlangle {1,0,0} rightrangle hspace{0.25in}vec j = leftlangle {0,1,0} rightrangle hspace{0.25in}vec k = leftlangle {0,0,1} rightrangle ]In two dimensional space there are two standard basis vectors,
[vec i = leftlangle {1,0} rightrangle hspace{0.25in}vec j = leftlangle {0,1} rightrangle ]Note that standard basis vectors are also unit vectors.
Warning
We are pretty much done with this section however, before proceeding to the next section we should point out that vectors are not restricted to two dimensional or three dimensional space. Vectors can exist in general n-dimensional space. The general notation for a n-dimensional vector is,
[vec v = leftlangle {{a_1},{a_2},{a_3}, ldots ,{a_n}} rightrangle ]and each of the ({a_i})'s are called components of the vector.
Unit 3 Basic Differentiationap Calculus Calculator
Because we will be working almost exclusively with two and three dimensional vectors in this course most of the formulas will be given for the two and/or three dimensional cases. However, most of the concepts/formulas will work with general vectors and the formulas are easily (and naturally) modified for general n-dimensional vectors. Also, because it is easier to visualize things in two dimensions most of the figures related to vectors will be two dimensional figures.
Unit 3 Basic Differentiationap Calculus Solver
So, we need to be careful to not get too locked into the two or three dimensional cases from our discussions in this chapter. We will be working in these dimensions either because it's easier to visualize the situation or because physical restrictions of the problems will enforce a dimension upon us.
